Distribution
As Dims were changed to Omegas their intensity decreased till all Dims vanished and instead a huge number of Omegas were created . It will be discussed later that number of time slices passed were not more than TN<200 and number of Omegas would not be more than N=10200 . Assume maximum number of Omegas to be N . This number could never be changed, except in a reverse process.
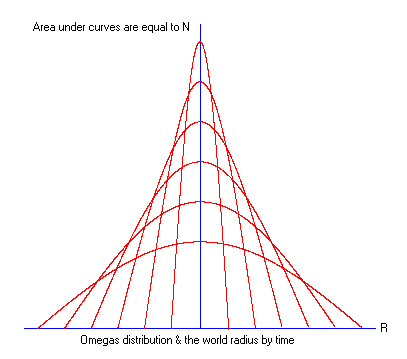
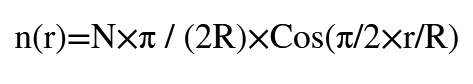
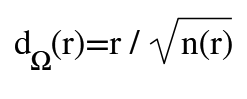
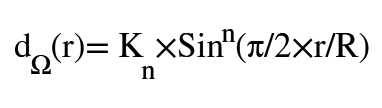
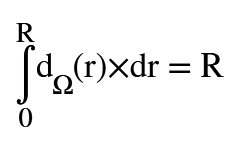

It is clear that the the world would be expanded by time and the force between Omegas makes them to go farther from each other and time would not be freeze at TN and also the world sized would not remain the same size as size at TN . Omegas as beads on rubber strings would change distance to each other by time , and the world size increases by time .
It is clear that all parts of the world would not the same speed of expansion . The Omegas near to center have very low speed , but the Omegas at the World edge (Horizon) would have maximum speed which could be defined as Light speed , same as beads on the rubber string.
An important result is defining a model for distribution of Omegas as time increases. Considering uniform probability of distribution in all possible directions , and also infinite sum of this distribution versus time makes the total distribution of Omegas as Normal Function .

This picture shows the distribution of Omegas is Normal Function but the area under each curve should remain to the original number of Omegas which is N .
Since Normal Function is not a handy formula for further calculations so it could be approximated by a cosine function .
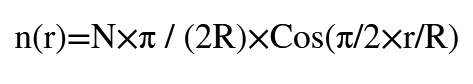
In this formula function n(r) is the total number of Omegas on surface of sphere with the radius (r).
Area under each curve remain constant and is equal to N.
Using this formula the approximate distance between successive Omegas could be found.
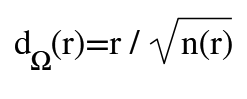
For uniform distribution of Omegas on surface of sphere with radius r the above formula is derived.
This shows distance between Omegas is minimum at center (r =0) and would be maximum at edge of the world (r =R) .
It should be considered that the shape of Omegas are not spherical it is disk type. Radius of disk is calculated by the above formula but the thickness if disk at the center could be calculated as
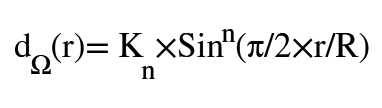
This is a formula for beads on a rubber string which Omegas have not equal distance is an increasing function.
Clearly the following formula should be accomplished by
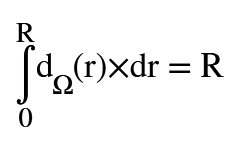
This would give the following result
